研究领域：代数几何与复几何，主要研究 Fano 流形、球簇、有理齐性空间等代数簇上的几何问题

  

  qifengli@sdu.edu.cn

 

 

2010年09月至2015年07月，中国科学院大学，基础数学，博士，导师：付保华

2006年09月至 2010年06月，山东大学，数学与应用数学，学士

2022年03月至今，山东大学，教授

2020年10月至2022年02月，韩国基础科学研究院，高级研究员

2015年08月至2020年09月，韩国高等研究院，博士后

山东大学齐鲁青年学者，山东大学杰出中青年学者，入选国家级青年人才

 

1.Baohua Fu, Qifeng Li: Rigidity of wonderful group compactifications under Fano deformations, to appear in Journal of Differential Geometry.

2.Jun-Muk Hwang, Qifeng Li: Recognizing the 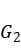-horospherical manifold of Picard number 1 by varieties of minimal rational tangents, Transformation Groups, 29 (2024), 143–178

3.Yifei Chen, Baohua Fu, Qifeng Li: Rigidity of projective symmetric manifolds of Picard number 1 associated to composition algebras, Épijournal Géom. Algébrique 2023, Special volume in honour of Claire Voisin, Article 4, 18 pp

4.Jun-Muk Hwang, Qifeng Li: Lines on holomorphic contact manifolds and a generalization of (2,3,5)-distributions to higher dimensions, Nagoya Mathematical Journal, 251 (2023), 652–668.

5.Qifeng Li: Fano deformation rigidity of rational homogeneous spaces of submaximal Picard numbers, Mathematische Annalen, 383 (2022), no. 1-2, 203-257.

6.Jun-Muk Hwang, Qifeng Li: Minimal rational curves and 1-flat irreducible G-structures, The Journal of Geometric Analysis, 2022, 32(6): Paper No. 179.

7.Jun-Muk Hwang*, Qifeng Li: Unbendable rational curves of Goursat type and Cartan type. Journal de Mathématiques Pures et Appliquées 155 (2021), 1-31.

8.Jun-Muk Hwang, Qifeng Li: Characterizing symplectic Grassmannians by varieties of minimal rational tangents. Journal of Differential Geometry 119 (2021), no. 2, 309-381.

1. 极小有理切簇理论与Fano流形的几何, 国家级，2023--2028

2. Fano流形的刻画与刚性,国家级，2022--2025